重置
序号 ID 年级 类型 来源 摘要 创建时间
26978 591265fbe020e7000878f6fc 高中 解答题 自招竞赛 已知向量 $\overrightarrow {OA} $ 与 $\overrightarrow {OB} $ 夹角为 $\alpha $，$\left| {\overrightarrow {OA} } \right| = 1 $，$ \left| {\overrightarrow {OB} } \right| = 2 $，$ \overrightarrow {OP} = \left({1 - t} \right)\overrightarrow {OA} $，$ \overrightarrow {OQ} = t\overrightarrow {OB} $，$ 0 \leqslant t \leqslant 1 $．$ \left| {\overrightarrow {PQ} } \right| $ 在 $ t = {t_0} $ 时取得最小值，问当 $ 0 < {t_0} < \dfrac{1}{5} $ 时，夹角 $ \alpha $ 的取值范围． 2022-04-17 20:58:59
26909 59128639e020e70007fbed6e 高中 解答题 自招竞赛 设 $S$ 是向量的集合，如果对 $S$ 中的元素 $\overrightarrow a $，$\overrightarrow a $ 的长度不小于其余所有向量之和的长度，那么称 $\overrightarrow a $ 是 $S$ 中的一个长向量．对于 $S = \left\{ {\overrightarrow {{a_1}} , \overrightarrow {{a_2}} , \cdots , \overrightarrow {{a_n}} } \right\}$，$n > 2$，已知 $S$ 中的每一个向量都为长向量，证明：$\overrightarrow {{a_1}} + \overrightarrow {{a_2}} + \cdots + \overrightarrow {{a_n}} = \overrightarrow 0 $． 2022-04-17 20:20:59
26601 591425ea1edfe2000949ce58 高中 解答题 高中习题 设 $\overrightarrow{a}=\left(1+\cos{\alpha},\sin{\alpha}\right)$，$\overrightarrow{b}=\left(1-\cos{\beta},\sin{\beta}\right)$，$\alpha\in(0,\pi)$，$\beta\in(\pi,2\pi)$，$\overrightarrow{a}$ 与 $x$ 轴正半轴的夹角为 $\theta_1$，$\overrightarrow{b}$ 与 $x$ 轴正半轴的夹角为 $\theta_2$，且 $\theta_1+\theta_2=\dfrac{\pi}{3}$，求 $\left|\overrightarrow{a}-\overrightarrow{b}\right|$ 的值． 2022-04-17 20:32:56
26178 597e96c6d05b90000b5e30fb 高中 解答题 高考真题 已知椭圆 $\Gamma$ 的方程为 $\dfrac {x^2}{a^2}+\dfrac {y^2}{b^2}=1(a>b>0)$，点 $P$ 的坐标为 $(-a,b)$． 2022-04-17 20:37:52
23966 59083883060a05000980b013 高中 解答题 高中习题 如图，在直角 $\triangle ABC$ 中，已知 $BC=a$．若长为 $2a$ 的线段 $PQ$ 以点 $A$ 为中点，问 $\overrightarrow{PQ}$ 与 $\overrightarrow{BC}$ 的夹角 $\theta$ 取何值时，$\overrightarrow{BP}\cdot\overrightarrow{CQ}$ 的值最大？并求出这个最大值． 2022-04-17 20:23:32
23127 590a82c56cddca0008610d0c 高中 解答题 高中习题 在半径为 $2$ 的球面上有三个点 $A$、$B$、$C$，求 $\overrightarrow{AB}\cdot\overrightarrow{AC}$ 的取值范围． 2022-04-17 20:41:24
23006 59112884e020e700094b08d3 高中 解答题 高中习题 在 $\triangle ABC$ 中，$A,B,C$ 所对的边分别为 $a,b,c$，$M$ 为 $AB$ 边上一点，$P$ 为直线 $CM$ 上一点，且 $\overrightarrow{CP}=\dfrac{\overrightarrow{CA}}{b\cos A}+\dfrac{\overrightarrow{CB}}{a\cos B}$，又已知 $\left|\overrightarrow{CM}\right|=\dfrac c2$，$a^2+b^2=2\sqrt 2ab$，求 $C$． 2022-04-17 20:35:23
22937 5925224b82e8bd0007792024 高中 解答题 高中习题 已知 $P$ 为 $\triangle ABC$ 内一点，求证：$S_A\overrightarrow{PA}+S_B\overrightarrow{PB}+S_C\overrightarrow{PC}=\overrightarrow 0$，其中 $S_{A}$，$S_{B}$，$S_{C}$ 分别是 $\triangle BPC$，$\triangle CPA$，$\triangle APB$ 的面积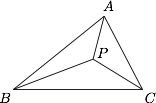． 2022-04-17 20:01:23
22867 595c8f676e0c650008344238 高中 解答题 高中习题 已知向量 $\overrightarrow a,\overrightarrow b$ 的夹角为 $\dfrac{\pi}3$，$\left|\overrightarrow a-\overrightarrow b\right|=5$，向量 $\overrightarrow c-\overrightarrow a,\overrightarrow c-\overrightarrow b$ 的夹角为 $\dfrac{2\pi}3$，$\left|\overrightarrow c-\overrightarrow a\right|=2\sqrt 3$，求 $\overrightarrow a\cdot \overrightarrow c$ 的最大值． 2022-04-17 20:23:22
22857 595c5303866eeb000bce0e44 高中 解答题 高中习题 已知 $A,B,C$ 是半径为 $1$ 的圆 $O$ 上的三点，$AB$ 为圆 $O$ 的直径，$P$ 为圆 $O$ 内（含圆周）一点，求 $\overrightarrow{PA}\cdot \overrightarrow{PB}+\overrightarrow{PB}\cdot\overrightarrow{PC}+\overrightarrow{PC}\cdot \overrightarrow{PA}$ 的取值范围． 2022-04-17 20:17:22
22617 59ba35d398483e0009c73180 高中 解答题 高中习题 在 $\triangle ABC$ 内取一点 $O$，设 $\overrightarrow e_1,\overrightarrow e_2,\overrightarrow e_3$ 分别是 $\overrightarrow{OA},\overrightarrow{OB},\overrightarrow{OC}$ 上的单位向量，求 $m=\left|\overrightarrow e_1+\overrightarrow e_2+\overrightarrow e_3\right|$ 的取值范围． 2022-04-17 20:54:19
22543 59fad8ee03bdb1000a37cb31 高中 解答题 自招竞赛 已知向量 $\overrightarrow a=\left(\cos{\dfrac{3x}{2}},\sin{\dfrac{3x}{2}}\right)$，$\overrightarrow b=\left(\cos{\dfrac x2},-\sin {\dfrac x2}\right)$，$x\in\left[0,\dfrac{\pi}{2}\right]$，求 $f(x)=\overrightarrow a\cdot \overrightarrow b-\left|\overrightarrow a+\overrightarrow b\right|$ 的最小值． 2022-04-17 20:10:19
21497 59719c58d3e6ac00094ed533 高中 解答题 自招竞赛 如图，$A,B$ 为椭圆 $\dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}=1(a>b>0)$ 和双曲线 $\dfrac{x^2}{a^2}-\dfrac{y^2}{b^2}=1$ 的公共顶点，$P,Q$ 分别为双曲线和椭圆上不同于 $A,B$ 的动点，且满足 $\overrightarrow{AP}+\overrightarrow{BP}=\lambda\left(\overrightarrow{AQ}+\overrightarrow{BQ}\right)$（$\lambda\in\mathbb R$，$|\lambda|>1$），求证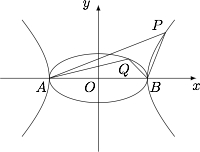： 2022-04-17 20:34:09
21467 591416320cbfff000adcab80 高中 解答题 高中习题 已知向量 $\overrightarrow a,\overrightarrow b$ 满足 $\left|\overrightarrow a+\overrightarrow b\right|=2m$，$\left|\overrightarrow a-\overrightarrow b\right|=2n$，求 $|\overrightarrow a|\cdot |\overrightarrow b|$ 的取值范围． 2022-04-17 20:18:09
20048 5cb5788f210b28021fc75650 高中 解答题 自招竞赛 已知 $O$ 是 $\triangle ABC$ 的外心，且 $3\overrightarrow{OA}+4\overrightarrow{OB} +5\overrightarrow{OC}=\overrightarrow{0} $，求 $ \cos\angle BAC$ 的值． 2022-04-17 19:08:56
20006 5cceb81c210b28021fc75dfc 高中 解答题 自招竞赛 已知函数 $f(x)=\dfrac{\sqrt{3}}{2}\sin 2x-\dfrac{1}{2}(\cos^2x-\sin^2x)-1,x\in\mathbf R$，将函数 $f(x)$ 的图像向左平移 $\dfrac{\pi}{6}$ 个单位后得到函数 $g(x)$ 的图像，设 $\triangle ABC$ 三个角 $A,B,C$ 的对边分别为 $a,b,c$． 2022-04-17 19:42:55
17282 5d19b708210b280220ed530a 高中 解答题 高中习题 在空间四边形 $ABCD$ 中，已知 $|A B|=3,|B C|=4,|C D|=5,|D A|=6$，则 $\overrightarrow{A C} \cdot \overrightarrow{B D}=$  ． 2022-04-17 19:54:30
17137 5e4ca977210b280d3782219c 高中 解答题 高考真题 已知抛物线 $C:x^2=-2py$ 经过点 $(2,-1)$．
（I）求抛物线 $C$ 的方程及其准线方程；
（II）设 $O$ 为原点，过抛物线 $C$ 的焦点作斜率不为 $0$ 的直线 $l$ 交抛物线 $C$ 于两点 $M,N$，直线 $y=-1$ 分别交直线 $OM,ON$ 于点 $A$ 和 $B$ 。求证：以 $AB$ 为直径的圆经过 $y$ 轴上的两个定点．		 2022-04-17 19:33:29
17103 5e3cd2f8210b286bd53192ac 高中 解答题 高考真题 已知曲线 $C:y=\dfrac{x^2}{2}$，$D$ 为直线 $y=-\dfrac{1}{2}$ 上的动点，过 $D$ 作 $C$ 的两条切线，切点分别为 $A,B$．
（1）证明：直线 $AB$ 过定点；
（2）若以 $E\left(0,\dfrac{5}{2}\right)$ 为圆心的圆与直线 $AB$ 相切，且切点为线段 $AB$ 的中点，求四边形 $ADBE$ 的面积．		 2022-04-17 19:15:29
16991 599165ca2bfec200011e1af4 高中 解答题 高考真题 已知向量 $\overrightarrow {a}=(\cos x,\sin x)$，$\overrightarrow {b}=(3,-\sqrt 3)$，$x \in (0,\pi)$． 2022-04-17 19:11:28
0.178994s