重置
序号 ID 年级 类型 来源 摘要 创建时间
16438 599165c12bfec200011e01ca 高中 解答题 高考真题 已知向量 $\overrightarrow a = \left( {\cos x, - \dfrac{1}{2}} \right)$，$\overrightarrow b = \left( {\sqrt 3 \sin x,\cos 2x} \right),x \in {\mathbb{R}}$，设函数 $f\left(x\right) = \overrightarrow a \cdot \overrightarrow b $． 2022-04-17 19:55:22
16429 599165c12bfec200011e018a 高中 解答题 高考真题 如图，椭圆的中心为原点 $O$，长轴在 $x$ 轴上，离心率 $e = \dfrac{\sqrt 2 }{2}$，过左焦点 ${F_1}$ 作 $x$ 轴的垂线交椭圆于 $A$，$A'$ 两点，$\left| {AA'} \right| = 4$．  2022-04-17 19:50:22
16427 599165c12bfec200011e00fd 高中 解答题 高考真题 设向量 $\overrightarrow a = \left( {\sqrt 3 \sin x,\sin x} \right)$，$\overrightarrow b = \left( {\cos x,\sin x} \right),x \in \left[ {0,\dfrac{\mathrm \pi} {2}} \right]$． 2022-04-17 19:50:22
16073 5f264523210b2865a6788600 高中 解答题 自招竞赛 正实数 $x,y,z,w$ 满足 $x\geqslant y\geqslant w$，且 $x+y\leqslant 2\left(w+z\right)$，求 $\frac{w}{x}+\frac{z}{y}$ 的最小值． 2022-04-17 19:28:19
16062 600e6524ba458b0009a55e10 高中 解答题 自招竞赛 给定一个凸四边形 $ABCD$，记 $\triangle BCD, \triangle CDA, \triangle DAB, \triangle ABC$ 的面积分别为 $S_A,S_B,S_C,S_D$．试在四边形 $ABCD$ 所在的平面上确定所有的点 $P$，使得$$S_A\cdot \overrightarrow{PA}+S_B\cdot \overrightarrow{PB}+S_C\cdot \overrightarrow{PC}+S_D\cdot \overrightarrow{PD}=\overrightarrow{0}.$$ 2022-04-17 19:23:19
16035 601f8e9525bdad000ac4d444 高中 解答题 自招竞赛 已知椭圆 $\Gamma: 3x^2+4y^2=12$ 和点 $Q(q,0)$，直线 $l$ 过 $Q$ 且与 $\Gamma$ 交于 $A,B$ 两点（可以重合）． 2022-04-17 19:08:19
16008 602e08bc25bdad000ac4d555 高中 解答题 自招竞赛 在平面直角坐标系 $xOy$ 中，$A$ 为椭圆 $\Gamma: \frac{x^2}{4}+y^2=1$ 上一点，$M$ 为线段 $OA$ 上的动点，过点 $M$ 作直线交椭圆 $\Gamma$ 于 $P,Q$ 两点．已知 $\overrightarrow{PM}=2\overrightarrow{MQ}$，求四边形 $OPAQ$ 面积的最大值． 2022-04-17 19:54:18
15956 6008f8218874860009b91fb5 高中 解答题 自招竞赛 已知等轴双曲线 $C:x^2-y^2=a^2$（$a>0$）上一定点 $P(x_0,y_0)$ 及双曲线 $C$ 上两动点 $A,B$ 满足 $(\overrightarrow{OA}-\overrightarrow{OP})\cdot (\overrightarrow{OB}-\overrightarrow{OP})=0$．（其中 $O$ 为坐标原点）． 2022-04-17 19:22:18
15576 59531967d3b4f900095c63f0 高中 解答题 高中习题 如图，已知扇形 $AOB$ 的圆心角为 $120^\circ$，$P$ 为弧 $AB$ 上一点，$\overrightarrow{OP}=x\overrightarrow{OA}+y\overrightarrow{OB}$．求 $x+y$ 的取值范围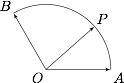． 2022-04-17 19:54:14
15567 5957ba13d3b4f90007b6fd54 高中 解答题 高中习题 已知 $O$ 为锐角三角形 $ABC$ 的外心，$A=\dfrac{\pi}3$，且 $\overrightarrow{OA}=x\overrightarrow{OB}+y\overrightarrow{OC}$，求 $2x-y$ 的取值范围． 2022-04-17 19:50:14
15297 5a0051f303bdb100096fbe17 高中 解答题 自招竞赛 向量 $ \overrightarrow a,\overrightarrow b$ 的夹角为 $\dfrac{\pi}3$，且 $\left|\overrightarrow a\right|=\left|\overrightarrow b\right|=1$． 2022-04-17 19:24:12
14964 621df585ea59ab000911898d 高中 解答题 高中习题 在平行四边形 $ABCD$ 中，$M,N$ 分别为边 $BC,CD$ 的中点，$\overrightarrow{AM}=\overrightarrow{a}, \overrightarrow{AN}=\overrightarrow{b}$，用 $\overrightarrow{a},\overrightarrow{b}$ 表示 $\overrightarrow{AC}$． 2022-04-17 19:22:09
14906 5908480a060a05000980b08c 高中 解答题 高中习题 已知 $\overrightarrow a$，$\overrightarrow b$ 是非零向量，构造集合 $P=\left\{\overrightarrow p\left|\overrightarrow p=t\overrightarrow a+\overrightarrow b,t\in\mathbb R\right.\right\}$．记 $P$ 中模最小的向量为 $T\left(\overrightarrow a,\overrightarrow b\right)$． 2022-04-17 19:48:08
14813 623c1f4eea59ab000a73de18 高中 解答题 高中习题 已知向量 $\overrightarrow{a}=(-2, -1)$，$\overrightarrow{b}=(t, 1)$，且 $\overrightarrow{a}$ 与 $\overrightarrow{b}$ 的夹角为钝角，求实数 $t$ 的取值范围． 2022-04-17 19:54:07
14812 623c20a5ea59ab0009118e99 高中 解答题 高中习题 如图，四边形 $ABCD$ 中，已知 $\overrightarrow{AD}=2\overrightarrow{BC}$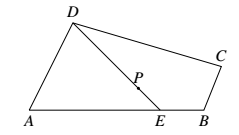． 2022-04-17 19:53:07
14811 623c255aea59ab0009118ec1 高中 解答题 高中习题 已知 $\odot O$ 内接 $\triangle ABC$，点 $D$ 为边 $BC$ 上一点，点 $E$ 为边 $AC$ 的中点，$AD$ 与 $BE$ 交于点 $P$，且 $\overrightarrow{BP}=4\overrightarrow{PE}$． 2022-04-17 19:52:07
14810 623c2658ea59ab0009118ec7 高中 解答题 高中习题 如图，向量 $\overrightarrow{OA}$，$\overrightarrow{OB}$ 的夹角为 $120^\circ$，$|\overrightarrow{OA}|=|\overrightarrow{OB}|=1$，点 $D$ 在以 $O$ 为圆心的圆弧 $AB$ 上运动，设 $\overrightarrow{OD}=x\overrightarrow{OA}+y\overrightarrow{OB}$，求 $x+y$ 的最大值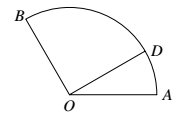． 2022-04-17 19:52:07
14809 623c2969ea59ab000a73de6a 高中 解答题 高中习题 已知向量 $\overrightarrow{a}=(-2, -1)$，$\overrightarrow{b}=(t, 1)$，且 $\overrightarrow{a}$ 与 $\overrightarrow{b}$ 的夹角为钝角，求实数 $t$ 的取值范围． 2022-04-17 19:51:07
14808 623c2a5eea59ab0009118ee6 高中 解答题 高中习题 已知点 $A(4,0)$，$B(4,4)$，$C(2,6)$，$O(0,0)$，求直线 $AC$ 与 $OB$ 交点 $P$ 的坐标． 2022-04-17 19:51:07
14807 623c2ceaea59ab000a73de85 高中 解答题 高中习题 在平面直角坐标系 $xOy$ 中，$A(1,0)$，$B(0,1)$，$C(2,5)$，$D$ 是 $AC$ 上的动点，满足 $\overrightarrow{AD}=\lambda\overrightarrow{AC}$，$\lambda\in\mathbb{R}$． 2022-04-17 19:50:07
0.187989s