如图,向量 $\overrightarrow{OA}$,$\overrightarrow{OB}$ 的夹角为 $120^\circ$,$|\overrightarrow{OA}|=|\overrightarrow{OB}|=1$,点 $D$ 在以 $O$ 为圆心的圆弧 $AB$ 上运动,设 $\overrightarrow{OD}=x\overrightarrow{OA}+y\overrightarrow{OB}$,求 $x+y$ 的最大值.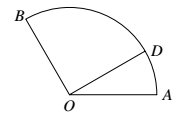

【难度】
【出处】
无
【标注】
【答案】
$2$
【解析】
略
答案
解析
备注