已知 $x+2y+\sqrt{xy}=2$,求 $x+3y$ 的取值范围.
【难度】
【出处】
无
【标注】
【答案】
$\left[\dfrac{12}7,3\right]$
【解析】
首先,$x\geqslant 0$,$y\geqslant 0$.否则$$x+2y+\sqrt{xy}\leqslant -2\sqrt{2xy}+\sqrt{xy}\leqslant 0,$$与已知条件矛盾.设 $a=\sqrt x$,$b=\sqrt y$,则原问题转化为已知 $a^2+2b^2+ab=2$,求 $a^2+3b^2$ 的取值范围.
再令 $a=r\cos\theta$,$b=\dfrac{1}{\sqrt 3}\cdot r\sin\theta$,$\theta\in\left[0,\dfrac{\pi}{2}\right]$,$r>0$,则所求代数式即 $r^2$,而$$r^2\cos^2\theta+\dfrac 23r^2\sin^2\theta+\dfrac 1{\sqrt 3}r^2\sin\theta\cos\theta=2,$$于是$$\dfrac 2{r^2}=\cos^2\theta+\dfrac 23\sin^2\theta+\dfrac{1}{\sqrt 3}\sin\theta\cos\theta=\dfrac 56+\dfrac 13\sin\left(2\theta+\dfrac{\pi}{6}\right),$$而$$\dfrac{\pi}{6}\leqslant 2\theta+\dfrac{\pi}{6}\leqslant \dfrac{7\pi}{6},$$于是可得$$\dfrac{12}{7}\leqslant r^2\leqslant 3,$$所求取值范围为 $\left[\dfrac{12}7,3\right]$.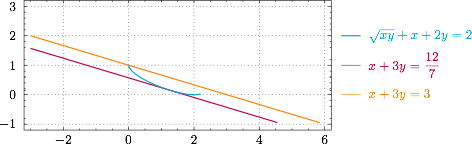
再令 $a=r\cos\theta$,$b=\dfrac{1}{\sqrt 3}\cdot r\sin\theta$,$\theta\in\left[0,\dfrac{\pi}{2}\right]$,$r>0$,则所求代数式即 $r^2$,而$$r^2\cos^2\theta+\dfrac 23r^2\sin^2\theta+\dfrac 1{\sqrt 3}r^2\sin\theta\cos\theta=2,$$于是$$\dfrac 2{r^2}=\cos^2\theta+\dfrac 23\sin^2\theta+\dfrac{1}{\sqrt 3}\sin\theta\cos\theta=\dfrac 56+\dfrac 13\sin\left(2\theta+\dfrac{\pi}{6}\right),$$而$$\dfrac{\pi}{6}\leqslant 2\theta+\dfrac{\pi}{6}\leqslant \dfrac{7\pi}{6},$$于是可得$$\dfrac{12}{7}\leqslant r^2\leqslant 3,$$所求取值范围为 $\left[\dfrac{12}7,3\right]$.

答案
解析
备注