已知椭圆 $C:x^2+2y^2=4$.
【难度】
【出处】
2014年高考北京卷(文)
【标注】
-
求椭圆 $C$ 的离心率;标注答案$\dfrac{\sqrt 2}2$解析根据题意,椭圆的长半轴长 $a=2$,短半轴长 $b=\sqrt 2$,因此半焦距 $c=\sqrt{a^2-b^2}=\sqrt 2$,离心率 $e=\dfrac ca=\dfrac{\sqrt 2}2$.
-
设 $O$ 为原点,若点 $A$ 在椭圆上,点 $B$ 在直线 $y=2$ 上,且 $OA\perp OB$,求线段 $AB$ 长度的最小值.标注答案$2\sqrt 2$解析设 $|OA|=r_1$,$|OB|=r_2$,点 $A$ 的坐标为 $A(r_1\cos\theta,r_1\sin\theta)$,其中 $\theta$ 表示以 $Ox$ 为始边,$OA$ 为终边的最小正角.
由 $OA\perp OB$ 可得点 $B$ 的坐标为 $B\left(r_2\cos\left(\theta\pm\dfrac{\pi}2\right),r_2\sin\left(\theta\pm\dfrac{\pi}2\right)\right)$.
由点 $A$ 在椭圆上,点 $B$ 在直线 $y=2$ 上,可得$$r_1^2\cos^2\theta+2r_1^2\sin^2\theta=4,r_2\sin\left(\theta\pm\dfrac{\pi}2\right)=2,$$因此\[\begin{split}|AB|^2&=r_1^2+r_2^2\\&=\dfrac{4}{\cos^2\theta+2\sin^2\theta}+\dfrac{4}{\cos^2\theta}\\&=\left(\dfrac{2}{\cos^2\theta+2\sin^2\theta}+\dfrac{2}{\cos^2\theta}\right)\cdot\left(\cos^2\theta+2\sin^2\theta+\cos^2\theta\right)\\&=4+\dfrac{2\cos^2\theta}{\cos^2\theta+2\sin^2\theta}+\dfrac{2\left(\cos^2\theta+2\sin^2\theta\right)}{\cos^2\theta}\\&\geqslant 8,\end{split}\]等号当 $\sin\theta=0$ 时取得.因此线段 $AB$ 长度的最小值为 $2\sqrt 2$.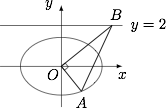
其他解法 直线 $AB$ 与圆 $x^2+y^2=2$ 相切.设 $B(t,2)$,$A(x_0,y_0)$,则 $OA:y=-\dfrac t2x$,与椭圆方程联立可得$$x_0^2=\dfrac{8}{t^2+2},$$因此\[\begin{split}|AB|^2&=|OA|^2+|OB|^2\\&=\left[1+\left(-\dfrac t2\right)^2\right]\cdot x_0^2+t^2+4\\&=t^2+6+\dfrac{4}{t^2+2}\geqslant 8,\end{split}\]等号当且仅当 $t=0$ 时取得.因此 $|AB|$ 的最小值为 $2\sqrt 2$.
题目
问题1
答案1
解析1
备注1
问题2
答案2
解析2
备注2