如图，四棱锥 $P - ABCD$ 中，底面是以 $O$ 为中心的菱形，$PO \perp $ 底面 $ABCD$，$AB = 2$，$\angle BAD = \dfrac{\mathrm \pi} {3}$，$M$ 为 $BC$ 上一点，且 $BM = \dfrac{1}{2}$，$MP \perp AP$．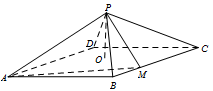
【难度】
【出处】
2014年高考重庆卷（理）
【标注】
-
求 $PO$ 的长；标注答案$ \dfrac{\sqrt 3 }{2}$．解析可通过向量法解决．将 $OP$ 的长度设出，通过 $\overrightarrow {MP}\cdot \overrightarrow {AP}=0$ 列出方程，解得 $PO$ 长．如图，连接 $AC$，$BD$，因 $ABCD$ 为菱形，则 $AC \cap BD = O $，且 $AC \perp BD$，
以 $O$ 为坐标原点，$\overrightarrow {OA}$，$\overrightarrow {OB}$，$\overrightarrow {OP} $ 的方向分别为 $x$ 轴，$y$ 轴，$z$ 轴的正方向，建立空间直角坐标系 $O - xyz$，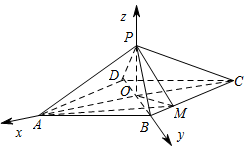
因为 $\angle BAD = \dfrac{{\mathrm \pi} }{3}$，故\[ OA = AB \cdot \cos \dfrac{\mathrm \pi} {6} = \sqrt 3 , OB = AB \cdot \sin \dfrac{{\mathrm \pi} }{6} = 1, \]所以\[ O\left( {0,0,0} \right) , A\left( {\sqrt 3 ,0,0} \right) , B\left( {0,1,0} \right) , C\left( { - \sqrt 3 ,0,0} \right) ,\]则根据空间向量的坐标运算可得\[ \overrightarrow {OB} = \left( {0,1,0} \right), \overrightarrow {BC} = \left( { - \sqrt 3 , - 1,0} \right). \]由 $BM = \dfrac{1}{2} $，$BC = 2 $，根据空间向量的数乘知\[\overrightarrow {BM} = \dfrac{1}{4}\overrightarrow {BC} = \left( { - \dfrac{\sqrt 3 }{4}, - \dfrac{1}{4},0} \right),\]从而根据向量加减法有\[\overrightarrow {OM} = \overrightarrow {OB} + \overrightarrow {BM} = \left( { - \dfrac{\sqrt 3 }{4},\dfrac{3}{4},0} \right),\]即 $M\left( { - \dfrac{\sqrt 3 }{4},\dfrac{3}{4},0} \right)$．
设 $P\left( {0,0,a} \right)$，$a > 0$，则\[\overrightarrow {AP} = \left( { - \sqrt 3 ,0,a} \right), \overrightarrow {MP} = \left( {\dfrac{\sqrt 3 }{4}, - \dfrac{3}{4},a} \right).\]因为 $MP \perp AP$，故 $\overrightarrow {MP} \cdot \overrightarrow {AP} = 0$，即 $- \dfrac{3}{4} + {a^2} = 0$，所以\[a = \dfrac{\sqrt 3 }{2} 或 a = - \dfrac{\sqrt 3 }{2} \left(舍去\right),\]即 $PO = \dfrac{\sqrt 3 }{2}$． -
求二面角 $A - PM - C$ 的正弦值．标注答案$\dfrac{{\sqrt {10} }}{5}$．解析可用向量法求二面角的余弦值，最后转化成正弦值．由（1）知\[ \overrightarrow {AP} = \left( { - \sqrt 3 ,0,\dfrac{\sqrt 3 }{2}} \right), \overrightarrow {MP} = \left( {\dfrac{\sqrt 3 }{4}, - \dfrac{3}{4},\dfrac{\sqrt 3 }{2}} \right), \overrightarrow {CP} = \left( {\sqrt 3 ,0,\dfrac{\sqrt 3 }{2}} \right), \]设平面 $APM$ 的法向量为 $\overrightarrow {n_1} = \left( {{x_1},{y_1},{z_1}} \right) $，平面 $PMC$ 的法向量为 $\overrightarrow {n_2} = \left( {{x_2},{y_2},{z_2}} \right) $，由\[\overrightarrow {n_1} \cdot \overrightarrow {AP} = 0,\overrightarrow {n_1} \cdot \overrightarrow {MP} = 0,\]得\[{\begin{cases}
- \sqrt 3 {x_1} + \dfrac{\sqrt 3 }{2}{z_1} = 0, \\
\dfrac{\sqrt 3 }{4}{x_1} - \dfrac{3}{4}{y_1} + \dfrac{\sqrt 3 }{2}{z_1} = 0 ,\\
\end{cases}}\]故可取 $\overrightarrow {n_1} = \left( {1,\dfrac{5\sqrt 3 }{3},2} \right)$，由\[\overrightarrow {n_2} \cdot \overrightarrow {MP} = 0,\overrightarrow {n_2} \cdot \overrightarrow {CP} = 0,\]得\[{\begin{cases}\dfrac{\sqrt 3 }{4}{x_2} - \dfrac{3}{4}{y_2} + \dfrac{\sqrt 3 }{2}{z_2} = 0,\\
\sqrt 3 {x_2} + \dfrac{\sqrt 3 }{2}{z_2} = 0 ,\\
\end{cases}}\]故可取 $\overrightarrow {n_2} = \left( {1, - \sqrt 3 , - 2} \right)$，从而法向量 $\overrightarrow {n_1} $，$\overrightarrow {n_2} $ 的夹角的余弦值为\[\cos \left\langle \overrightarrow {n_1} ,\overrightarrow {n_2} \right \rangle = \dfrac{{\overrightarrow {n_1} \cdot \overrightarrow {n_2} }}{{ \left|\overrightarrow {n_1} \right| \cdot \left |\overrightarrow {n_2} \right |}} = - \dfrac{{\sqrt {15} }}{5},\]则所求二面角的余弦值的绝对值为 $\dfrac{{\sqrt {15} }}{5}$，根据同角三角函数的基本关系可得所求二面角 $A - PM - C$ 的正弦值为 $\dfrac{{\sqrt {10} }}{5}$．
题目
问题1
答案1
解析1
备注1
问题2
答案2
解析2
备注2
