查看数据源：599165c32bfec200011e076d
若以直角坐标系的原点为极点，$x$ 轴的非负半轴为极轴建立极坐标系，则线段 $y=1-x\left(0 \leqslant x \leqslant 1\right)$ 的极坐标方程为 \((\qquad)\)
A: $\rho = \dfrac{1}{\cos \theta + \sin \theta} $，$ 0 \leqslant \theta \leqslant \dfrac{{\mathrm{\mathrm \pi} }}{2}$
B: $\rho = \dfrac{1}{\cos \theta + \sin \theta} $，$ 0 \leqslant \theta \leqslant \dfrac{{\mathrm{\mathrm \pi} }}{4}$
C: $\rho = {\cos \theta + \sin \theta}$，$0 \leqslant \theta \leqslant \dfrac{{\mathrm{\mathrm \pi} }}{2}$
D: $\rho = {\cos \theta + \sin \theta} $，$ 0 \leqslant \theta \leqslant \dfrac{{\mathrm{\mathrm \pi} }}{4}$
【难度】
【出处】
2014年高考江西卷（理）
【标注】
  • 知识点
    >
    解析几何
    >
    极坐标方程
  • 题型
    >
    解析几何
【答案】
A
【解析】
由极坐标与直角坐标的互化公式带入，整理即可，然后需要注意，在 $0\leqslant x\leqslant 1$ 时，$\theta$ 的取值范围．由极坐标与直角坐标的互化公式得直线 $y=1-x$ 的极坐标方程为\[\rho\sin \theta=1-\cos \theta,\]即 $\rho = \dfrac{1}{\cos \theta + \sin \theta} $．
在直角坐标系画出原线段的图象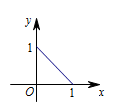：所以其对应的极坐标方程中极角的范围是 $\left[ 0 ,\dfrac{{\mathrm{\mathrm \pi} }}{2}\right]$．
综上，线段 $y=1-x\left(0 \leqslant x \leqslant 1\right)$ 的极坐标方程为 $\rho = \dfrac{1}{\cos \theta + \sin \theta} $，$ 0 \leqslant \theta \leqslant \dfrac{{\mathrm{\mathrm \pi} }}{2}$．
题目 答案 解析 备注
0.113704s